If you have been looking at this site, you may have wondered "why all these Cayley diagrams? What is the point?" This page tries to answer that.
There are three reasons I like creating Cayley diagrams:
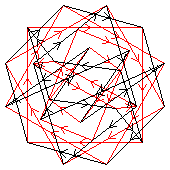
To illustrate the above, here are some Cayley diagrams for the binary tetrahedral group, isomorphic to SL(2,3), in the order in which I drew them.
Given a presentation for a group, it is easy, if tedious, to draw some sort of valid Cayley diagram for it, if you don't mind the lines crossing. My first version for SL(2,3), as seen on the left, is a good example of this. As usual if you do no planning, the result looks like a magpie's nest (right). It contributes nothing to understanding, is not pretty, and was not a challenge to draw.
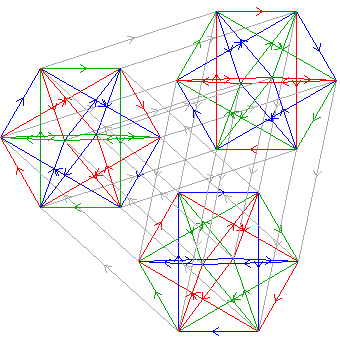My second version, to the left, was much better, particularly with regard to
understanding at least a little of the structure of the group. Each of the three
coloured clusters is a Cayley diagram for Q8. The whole diagram makes it clear
that SL(2,3) can be regarded as Q8 ⋊ C3, with the C3 permuting the generators
of the Q8, the permutation being (red blue green), or, using the standard
notation for the quaternion group Q8, (i j k),(-i -j -k).
Incidentally, my Cayley diagrams are all generated by perl programs. The program to draw this diagram is pleasingly short.
My third version, to the right, uses toll bean notation, as explained in
How to Build Groups: Toll-bean Extensions. I no
longer think that toll bean extensions are a good way to portray covers of groups.
My fourth version, to the left, is drawn on a torus. It is pretty in its
way, but does little, at least for me, to clarify the structure of SL(2,3). All
that can be said for it is that it is better than the magpie-nest version and the
toll-bean version above. The motivation to create this, and many other
Cayley diagams drawn on toruses and other
2-manifolds, was the challenge of drawing them
without the arcs crossing.
The fifth version, to the right, was sent to me by an anonymous contributor.
I like this one. I do not know how he found it; his understanding of the group must
be better than mine. ... Now that I think about it, I can see a way he might have
found it. Just as SL(2,3) is a double cover of A4 (A4 is what you get if you remove
the orange triangles from the diagram above right), so this diagram is a double cover
of the A4 diagram. The triangles have become hexagons which go round twice, and the
red edges have become quadilaterals which go back and forth twice.
Cayley diagrams for many small groups can be drawn without crossings on a plane; or equivalently, on a sphere. Many, like the one on the right for A5, would look more regular on a sphere, but have been flattened onto a plane. In fact any finite subgroup of SO(3) can be drawn on a sphere, and therefore on a plane.
The smallest oriented 2-manifold on which a Cayley diagram for a group can be drawn
(with a suitable choice of generators) without any arcs crossing is known as the
genus of the group. If a sphere will do, the genus is 0, otherwise if a torus
will do it is 1, etc.
Some more Cayley diagrams
Some more pages on groups
Copyright N.S.Wedd 2009